Isometric projection of Cylinder
Sample Problem:
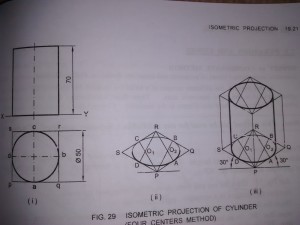
Draw the Isometric projection of Cylinder of base 50 mm diameter and 70 mm height when it rests with its base on HP.
Solution for Isometric projection of Cylinders using BOX method:
- Fig.1 Draw the orthographic projections of the cylinder. Enclose the circle in the top view in a square pqrs. Mark mid-points of pq, qr, rs and sp as a,b,c and d respectively in the top view.
- Fig.2 draw the isometric projection of the square as rhombus PQRS as shown.
- Mark the mid-points of PQ, QR, RS and SP as A, B, C and D respectively.
- Join P with B and C which are the mid-points of the opposite sides of the rhombus, Similarly join R with A and D.
- With P as center and PC as radius draw an arc CB. Similarly, R as center and RA as radius draw an arc AD. Lines PC and RD intersect at O1.
- With O1 as center and O1 D as radius draw an arc DC.
- Similarly, with O2 (intersection of RA and PB) as center and O2B as radius draw an arc BA. Thus complete the ellipse to represent the base circle.
- Fig 3. Draw the Isometric projection of the box, taking its height = Height of the cylinder in isometric length. Construct another ellipse on the top surface of the box. Draw two common tangents to the ellipses. Complete the isometric projection.