Construction of Hyperbola
Sample Problem 1:
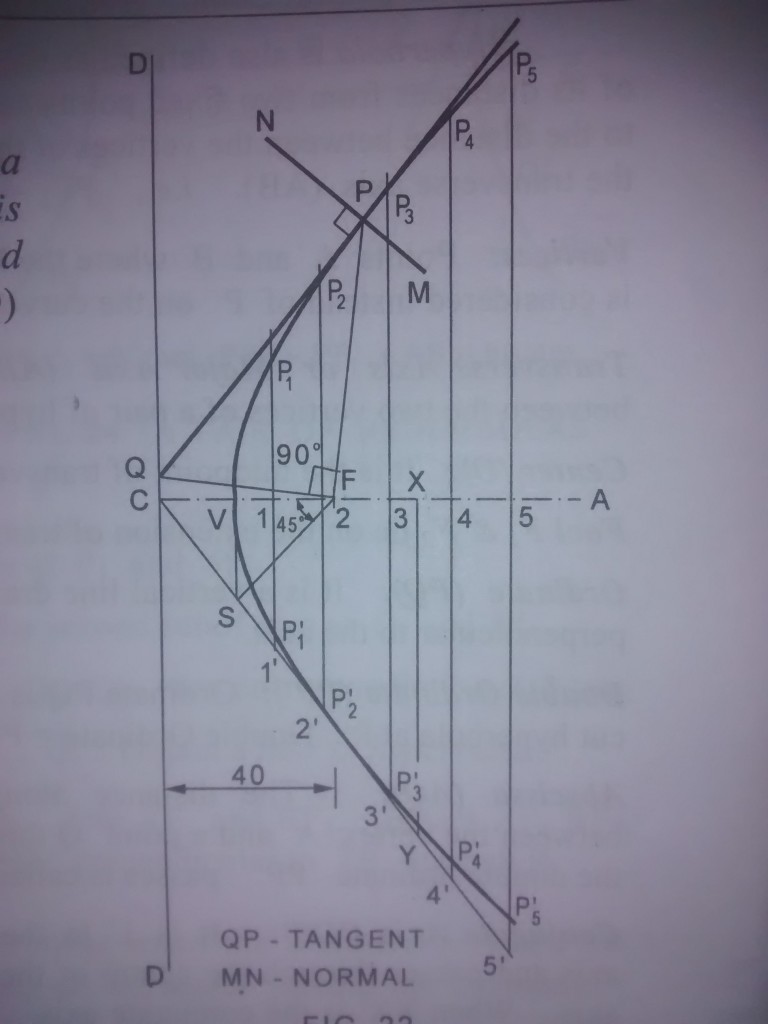
Construct a hyperbola when the distance between the focus and the directrix is 40mm. The eccentricity is 4/3. Draw a tangent and normal at any point on the hyperbola.
Steps for Construction of Hyperbola:
- Draw directrix DD. At any point C on it draw CA perpendicular to DD to represent the axis.
- Mark F the focus , Such that CF=40mm.
- e=4/3. So construct right-angled ΔCXY such that XY/CX = 4units/3units (X is any point on axis).
- From F draw a 45º line to cut CY at S.
- From S erect vertical to intersect CF at V, the Vertex. Now SV = FV.
- From similar Δs CXY and CVS,
SV/CV = YX/CX = FV/CV = 4/3. - Along CA mark 1,2,3,4,5 at approximately equal intervals.
- Through 1,2,3,4,5 erect verticals to intersect CY (Produced if necessary) at 1′,2′,…,5′ respectively.
- 11′ as radius and F as center draw two arcs on either side of the axis to cut the vertical line drawn through 1 at P1 and P1′.
- Repeat the above. Obtain P2 and P2′,…,P5 and P5′ corresponding to 2,3,4 and 5 respectively. Draw a smooth hyperbola through P5,P4,…,P1,V,P1′,…,P5′.
- Tangent and Normal: Join PF. At F , draw a perpendicular to PF to meet CD at Q. Join PQ, the tangent. Draw NM perpendicular to PQ. NM is the normal.
Construction of Hyperbola Video Materials
For more Click below ⇓
GE6152 Engineering Graphics Video Lectures